Depth First Search Algorithm to Compute the Smallest String Star

Time：2020-09-09 13:16:32
Class：Weblog
Read：16

Given the root of a binary tree, each node has a value from 0 to 25 representing the letters ‘a’ to ‘z’: a value of 0 represents ‘a’, a value of 1 represents ‘b’, and so on.

Find the lexicographically smallest string that starts at a leaf of this tree and ends at the root.

(As a reminder, any shorter prefix of a string is lexicographically smaller: for example, “ab” is lexicographically smaller than “aba”. A leaf of a node is a node that has no children.)

Example 1:
Input: [0,1,2,3,4,3,4]
Output: “dba”

Example 2:
Input: [25,1,3,1,3,0,2]
Output: “adz”

Example 3:
Input: [2,2,1,null,1,0,null,0]
Output: “abc”

Note:
The number of nodes in the given tree will be between 1 and 8500.
Each node in the tree will have a value between 0 and 25.

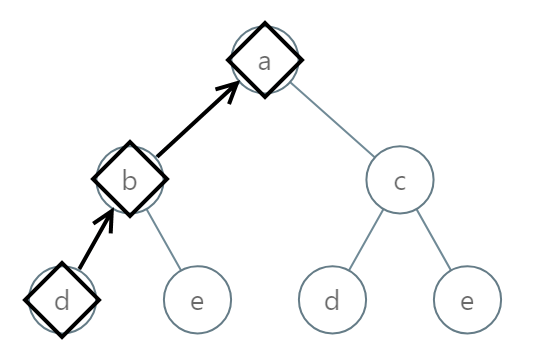
Recursive Depth First Search to Compute the Smallest String Starting From Leaf

We can start from the Root, perform a recursive DFS (Depth First Search) algorithm, and pass the string along down to the leaves. When we reach a leaf node, we construct the final string, and store the smallest one (alphabetically).

All nodes are visisted exactly once. When we finish searching the tree, we have the answer. The time complexity is O(N). In this particular case, the DFS is also the bruteforce algorithm.

/**
 * Definition for a binary tree node.
 * struct TreeNode {
 *     int val;
 *     TreeNode *left;
 *     TreeNode *right;
 *     TreeNode() : val(0), left(nullptr), right(nullptr) {}
 *     TreeNode(int x) : val(x), left(nullptr), right(nullptr) {}
 *     TreeNode(int x, TreeNode *left, TreeNode *right) : val(x), left(left), right(right) {}
 * };
 */
class Solution {
public:
    string smallestFromLeaf(TreeNode* root) {
        dfs(root, "");
        return ans;
    }
private:
    string ans = "";
    void dfs(TreeNode* root, string cur) {
        if (!root) return;
        if ((root->left == nullptr) && (root->right == nullptr)) {
            cur = (char)(97 + root->val) + cur;
            if ((ans == "") || (cur < ans)) {
               ans = cur; 
            }
            return;
        }
        dfs(root->left, (char)(97 + root->val) + cur);
        dfs(root->right,(char)(97 + root->val) + cur);
    }
};

/**
 * Definition for a binary tree node.
 * struct TreeNode {
 *     int val;
 *     TreeNode *left;
 *     TreeNode *right;
 *     TreeNode() : val(0), left(nullptr), right(nullptr) {}
 *     TreeNode(int x) : val(x), left(nullptr), right(nullptr) {}
 *     TreeNode(int x, TreeNode *left, TreeNode *right) : val(x), left(left), right(right) {}
 * };
 */
class Solution {
public:
    string smallestFromLeaf(TreeNode* root) {
        dfs(root, "");
        return ans;
    }
private:
    string ans = "";
    void dfs(TreeNode* root, string cur) {
        if (!root) return;
        if ((root->left == nullptr) && (root->right == nullptr)) {
            cur = (char)(97 + root->val) + cur;
            if ((ans == "") || (cur < ans)) {
               ans = cur; 
            }
            return;
        }
        dfs(root->left, (char)(97 + root->val) + cur);
        dfs(root->right,(char)(97 + root->val) + cur);
    }
};

Depth First Searching Algorithm using Stack

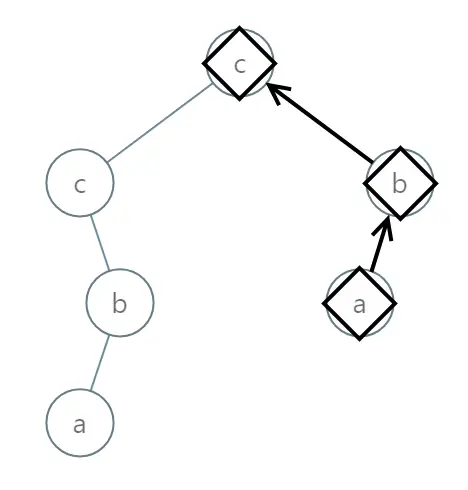
The Recursion uses implicit stacks. The compiler generates the calling stacks. The recursion code usually is more clean and concise. We can also use a stack to manually emulate the recursive calls.

/**
 * Definition for a binary tree node.
 * struct TreeNode {
 *     int val;
 *     TreeNode *left;
 *     TreeNode *right;
 *     TreeNode() : val(0), left(nullptr), right(nullptr) {}
 *     TreeNode(int x) : val(x), left(nullptr), right(nullptr) {}
 *     TreeNode(int x, TreeNode *left, TreeNode *right) : val(x), left(left), right(right) {}
 * };
 */
class Solution {
public:
    string smallestFromLeaf(TreeNode* root) {
        if (!root) return "";
        stack<pair<TreeNode*, string>> st;
        st.push({root, ""});
        string ans = "";
        while (!st.empty()) {
            auto p = st.top();
            st.pop();
            if (p.first->right) {
                st.push({p.first->right, (char)(97 + p.first->val) + p.second});
            }            
            if (p.first->left) {
                st.push({p.first->left, (char)(97 + p.first->val) + p.second});
            }
            if ((p.first->left == nullptr) && (p.first->right == nullptr)) {
                string v = (char)(97 + p.first->val) + p.second;
                if ((ans == "") || (v < ans)) {
                    ans = v;   
                }                
            }
        }
        return ans;
    }
};

/**
 * Definition for a binary tree node.
 * struct TreeNode {
 *     int val;
 *     TreeNode *left;
 *     TreeNode *right;
 *     TreeNode() : val(0), left(nullptr), right(nullptr) {}
 *     TreeNode(int x) : val(x), left(nullptr), right(nullptr) {}
 *     TreeNode(int x, TreeNode *left, TreeNode *right) : val(x), left(left), right(right) {}
 * };
 */
class Solution {
public:
    string smallestFromLeaf(TreeNode* root) {
        if (!root) return "";
        stack<pair<TreeNode*, string>> st;
        st.push({root, ""});
        string ans = "";
        while (!st.empty()) {
            auto p = st.top();
            st.pop();
            if (p.first->right) {
                st.push({p.first->right, (char)(97 + p.first->val) + p.second});
            }            
            if (p.first->left) {
                st.push({p.first->left, (char)(97 + p.first->val) + p.second});
            }
            if ((p.first->left == nullptr) && (p.first->right == nullptr)) {
                string v = (char)(97 + p.first->val) + p.second;
                if ((ans == "") || (v < ans)) {
                    ans = v;   
                }                
            }
        }
        return ans;
    }
};

During the code interviews, if you are asked this question, it is better to go with the Recursion. But you can follow up with the non-recursive/iterative approach.

–EOF (The Ultimate Computing & Technology Blog) —

Recommend：
C++ Coding Reference: is_sorted_until() and is_sorted()
Two Rectangles Overlap Detection Algorithm in C++
C++ Coding Reference: next_permutation() and prev_permutation()
C++ Coding Reference: count and count_if
How to Reorder Data in Log Files using the Custom Sorting Algori
C++ Coding Reference: std::accumulate() and examples
C++ Coding Reference: sort() and stable_sort()
The C++ Function using STL to Check Duplicate Elements/Character
How to Find Positive Integer Solution for a Given Equation using
How to Implement the instanceof in Javascript?

Comment list

Comment add: Name：　Check：